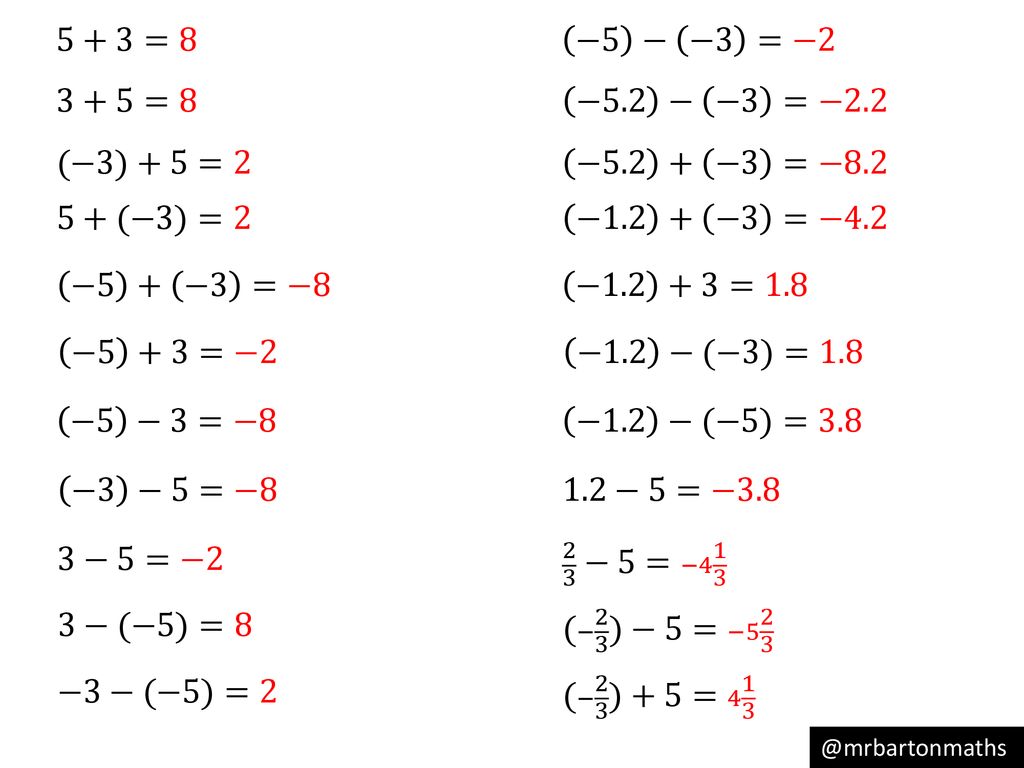
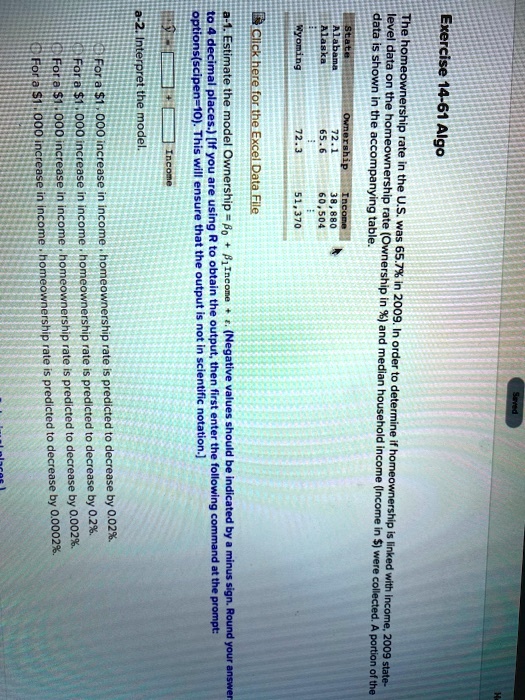
３−５ 277740

Systems Of Linear Equations Gaussian Elimination Studypug

 Ex 121, 7 Simplify (ii) (3^(−5 ) × 〖10〗^(−5 ) × 125)/(5^(−7 ) × 6^(−5) ) (3^(−5 )× 〖10〗^(−5 ) × 125)/(5^(−7 ) × 6^(−5) )= 3^(−5)×Example 5 > 3, x ≤ 4, x y ≥ 9 (i) Inequalities which do not involve variables are called numerical inequalities For example 3 < 8, 5 ≥ 2 (ii) Inequalities which involve variables are called literal inequalities For example, x > 3, y ≤ 5, x – y ≥ 0 (iii) An inequality may contain more than one variable and it can be linear , quadratic or cubic etc For eaxmple , 3x – 2 < 0

３−５

３−５- (𝑃(𝑥)) = 3𝑎 − 𝑏 = 3(3) − 2 = 7(V) El número de términos es Nº de términos =grado 1 =71=8 (V) El grado del término 3x3b−c es 5 Es decir 3(2) − 𝑐 = 5 → 𝑐 = 1 Finalmente 𝑎 𝑏 𝑐 = (3)(2)(1) = 6 (V) 8 Sean P(x) y Q(x) dos polinomios definidos por3 (0), ( 4), 1 , (3),f − 5 Determine whether each of the following equations defines y as a function of x (Do not graph) 55 x −5y = 56 x 3= y2 57 3x2 y = 58 x3x4 −2xy =5 59 7x − y4 = 5 60 =3x2 y2 16 61 x3 −2y = 6 62 5x −3y = 63 yx3 =5 64 y3 = −7x 65 y x− =2 66 x y =3 4 Exercise Set 11 An Introduction to Functions 4 University of Houston Department of

Probability Theory Definition Examples Facts Britannica

3 (2−5i)− 1 6 (8−2i) = 2 3 − 5 3 i− 8 6 2 6 i = 2 3 − 5 3 i− 4 3 1 3 i = 2 3 − 4 3 − 5 3 i 1 3 i = − 2 3 − 4 3 i which we could also write as −2 3 (12i) Click on the green square to returnUnit 2 The derivative of a function f is given by f′ (x)=01xe025x At what value of x for x>0 does the line tangent to the graph of f at x have slope 2 ?(a) 12,18 (b) 3,2 (c) 2,3 (d) −6,−9 (e) −24,−27 (f) −24,−36 In Exercises 29

 Find (2/3)^2 ×(2/5)^(−3) × (3/5)^2 (2/3)^2 ×(2/5)^(−3) × (3/5)^2 = (2/3)^2 ×(5/2)^3 × (3/5)^2 = 2^2/3^2 × 5^3/2^3 × 3^2/5^2 = 𝟓/𝟐 Evaluate (i) ∛16week Lesson 13 (8week Lesson 10) Solving Quadratic Equations by Completing the Square 5 c 𝑥24𝑥13=0 d3𝑥2=6𝑥−1 d A 3𝑥2 3 12𝑥 3 −39 3 =0 3𝑥 2 3 =6𝑥 3 −1 3 3𝑥2 3X x x x = − − 3 5 72 17 2 2 12 2 2 1 tan 3 15 315 2 !

３−５のギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	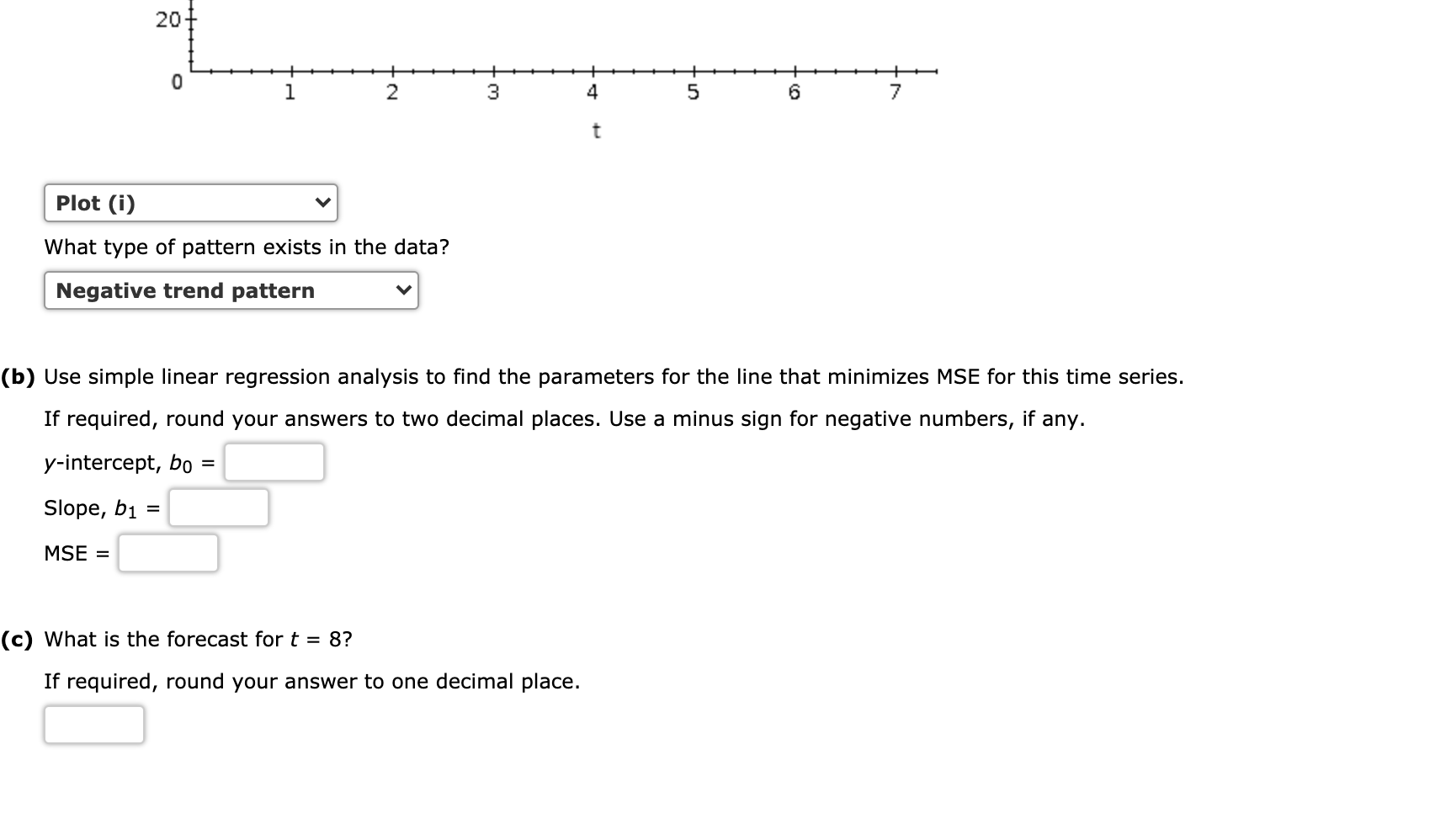Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	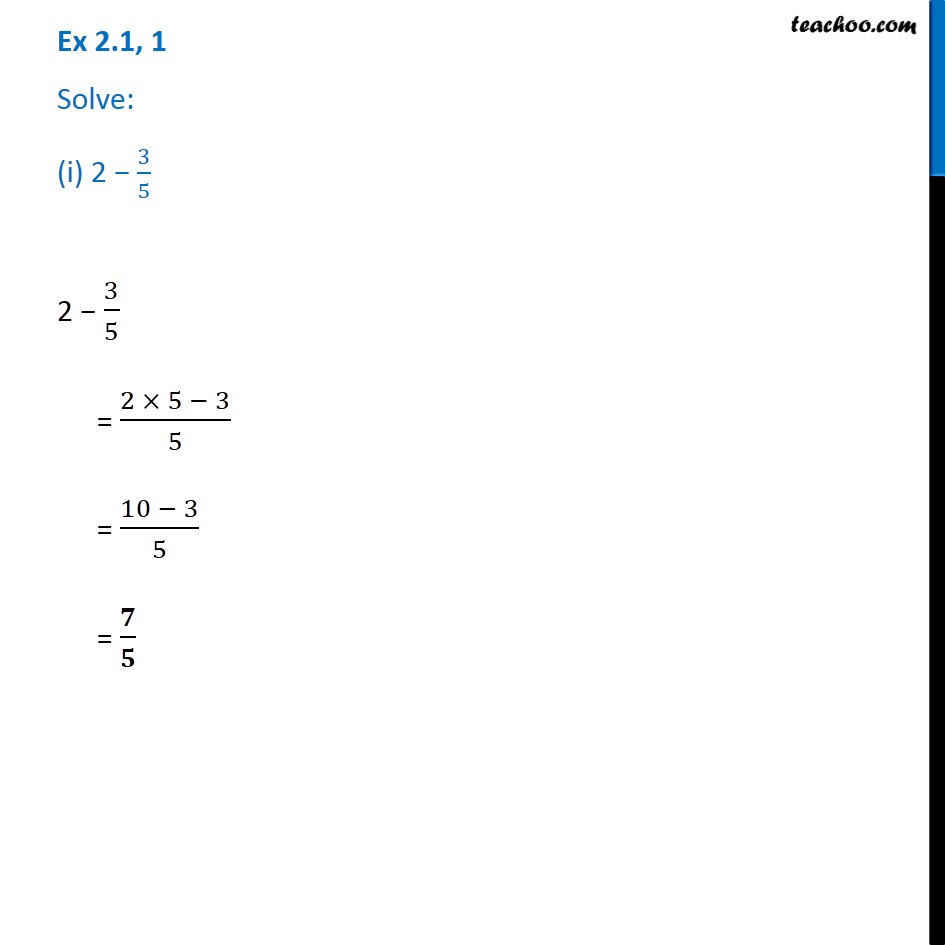Faculty Math Illinois Edu	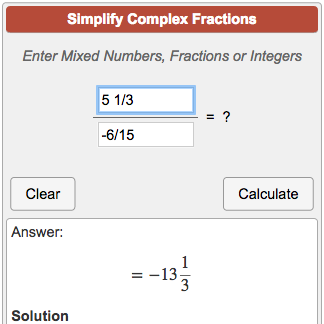Faculty Math Illinois Edu
Faculty Math Illinois Edu	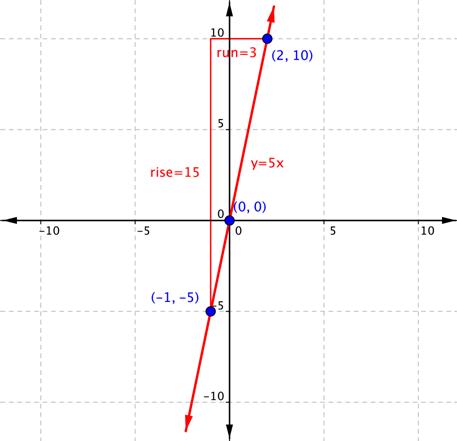Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	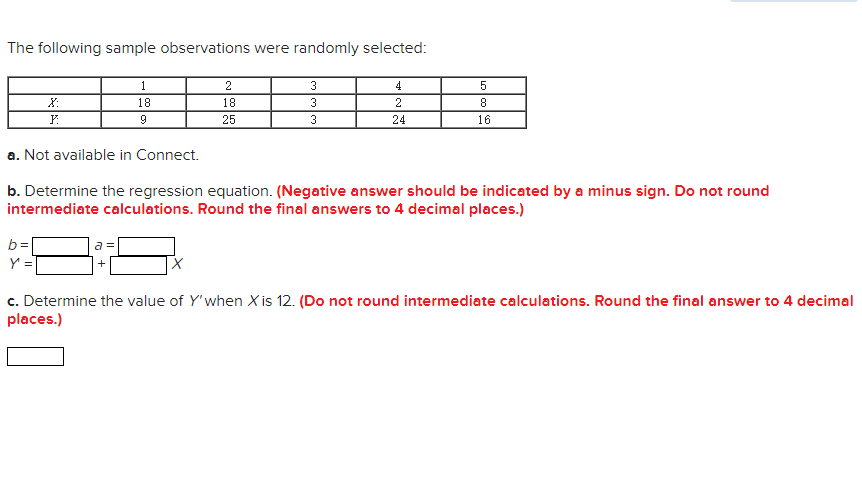Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	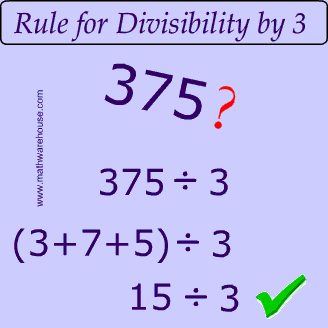Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	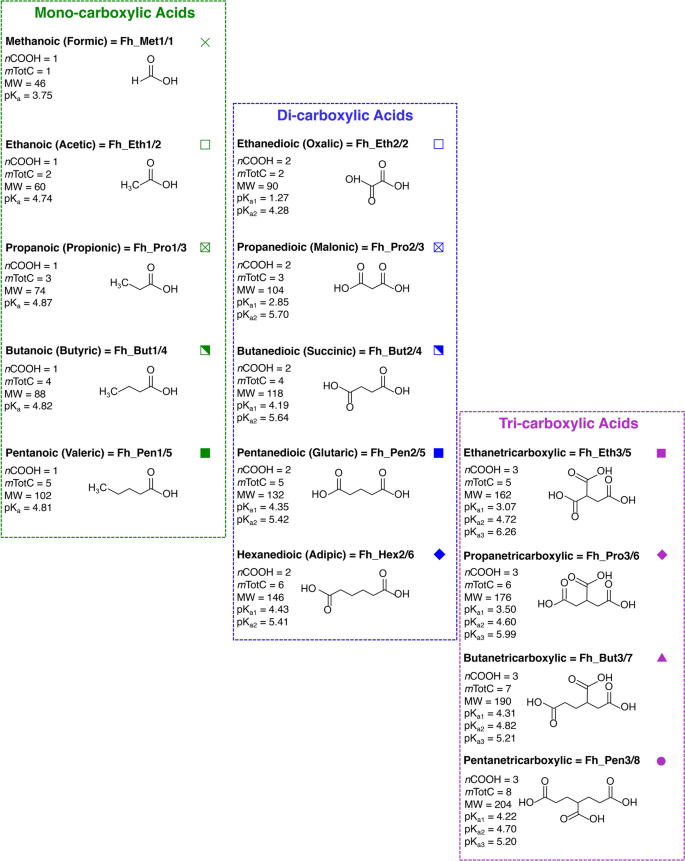Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu	Faculty Math Illinois Edu
Faculty Math Illinois Edu	Faculty Math Illinois Edu

4(4) 5(− 2) = 3 For this problem, you will use the substitution method Substitute x = − 2 and y = 4 into the equation 16 (− 10) = 3 6 = 3 Evaluate The statement is not true, so (− 2, 4) is not a solution to the equation 4y 5x = 3 Answer (−2, 4) is not a solution to the equation 4y 5x = 3A 11 = 12 − 5 = 7, a 12 = − (9 10) = − 19, a 13 = − 3 − 8 = − 11 a 21 = − (− 3 2) = 1, a 22 = 3 − 4 = − 1, a 23 = − (− 1 2) = − 1 a 31 = 5 − 8 = − 3, a 32 = − (− 5 − 6) = 11, a 33 = 4 3 = 7